
Robert Berman
Professor of Mathematics
Wallenberg Scholar
Institution:
Chalmers University of Technology
Research field:
Analytical aspects of complex algebraic and differential geometry
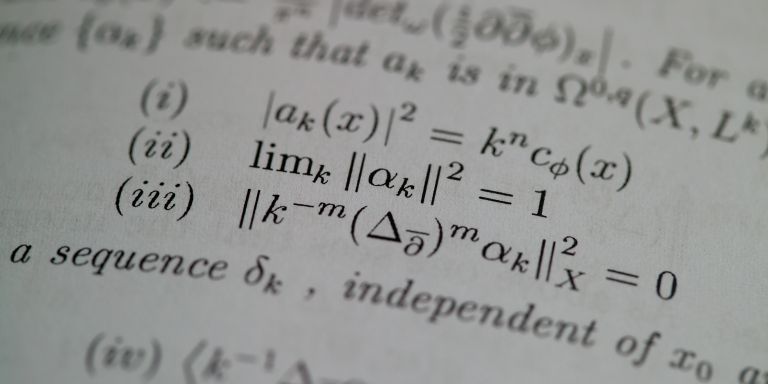

Wallenberg Scholar
Institution:
Chalmers University of Technology
Research field:
Analytical aspects of complex algebraic and differential geometry
Robert Berman is clearly a cheerful and enthusiastic person who claims that he has the world's most enjoyable job.
"It is pure adventure to be doing this job. I leap up out of bed every day, that's how much fun it is."
In his bookcase in his office at Chalmers in Gothenburg, there is almost as much poetry as mathematics. There are volumes by Gunnar Ekelöf, Karin Boye, Gustaf Fröding and Tomas Tranströmer, among others. He says that he likes literature, but also says that the honest answer to the question as to why there is poetry in his bookcase is that he was forced to clean out some at home.
"But the fact of the matter is that when I need to take a break at work, it is so nice to read a little poetry, and so it's appropriate to have some poetry here."
A basic characteristic that Robert Berman has is that he really wants to understand why things are as they are, and function the way they do. In other words, he is one of those people who have never contented himself with a because. That he ended up in mathematics was not at all self-evident. As a teenager, he was gripped by existential questions, and after high school he began studying philosophy. What captured his interest above all was the philosophy of science, but because he felt that philosophers were rather woolly, that they talked about science without really knowing what they were talking about, he began studying physics.
"During the first semester of the physics course, you studied mathematics and somewhere along the line there I got stuck. I thought it was great fun and noticed that I got into a "flow" when I worked with mathematical problems. Mathematics is so beautiful. When you understand something new, it's an aesthetic experience. So it turned out to be mathematics instead of physics."
Robert Berman tells us that you can distinguish between roughly two types of mathematician, and this is due to how the brain works. The left hemisphere is logical and analytical, that is, more algebraic. The right hemisphere is artistic and creative, that is, more geometric.
"In algebra, your reasoning goes step by step and that means you can easily lose track of the big picture. Instead, I have always been attracted by the geometric aspects of mathematics. My strength lies in being good at the big picture, that I have good intuition about what I would like to come up with, and where I am going."
One type of mathematics that he does is about explaining in general terms how collective, large-scale phenomena arise out of complex microscopic structures. A classic example of this type of mathematics is a water flow: even if the movements of the individual water molecules are chaotic, an orderly, large-scale structure arises.
But water is not the focus of Robert Berman's research these days. As a Wallenberg Academy Fellow, he instead intends to deepen his studies of the mathematical aspects of gravitation. One of the objectives is to develop a model where space–time geometry emerges as a macroscopic phenomenon out of an underlying microscopic structure that can be described with the help of complex algebraic geometry – a part of modern mathematics in which there has been substantial progress in recent years.
Isaac Newton claimed that the Earth travels in its orbit around the Sun due to its own speed in combination with the Sun's gravitational force. Albert Einstein came to the conclusion that this was not quite how things went. Einstein described instead gravitation as a manifestation of the curvature of space-time, that is, the geometry of space-time. To use a metaphor, one could imagine the Sun as a bowling ball that you place on a mattress which then creates a curvature in the entire mattress. When the Earth comes into the curvature created in space by the Sun, the Earth is influenced by the curvature and begins to follow it.
“If we were to look into this curvature with a hugely powerful magnifying glass, in physics one would expect that it is rather coarse and consists of different structures which together form the curvature. What I am trying to do is to describe how the small pieces together create the curvature. The challenge is thus to understand this global geometric structure, mathematically describe it, and thus provide an explanation as to why the universe looks the way it does.”
Today there is no microscopic theory of gravitation and this is precisely what attracts Robert Berman. The adventure aspect is important in his work. The exploration of an exciting world that he can only barely glimpse inspires him. He describes it as moving around in a dark room and vaguely sensing that there are things in the room, and then suddenly you find the light switch and then everything emerges in an explanatory light, and you see how everything hangs together at one and the same time.
"The most important thing is that it gives me time to do research. I simply get paid to think about these things and to interact with my doctoral students and colleagues. The grant also gives me the opportunity to recruit talented people who can broaden what we are doing and in this way we can build up a creative and innovative environment."
His research may also lead to new mathematical understanding of other complex systems.
“The fantastic thing is that the same type of equations that I am using to understand the universe's geometric structure can also be used, for example, to explain how warm and cold fronts form, and how signals can be sampled and compressed.”
Text Anders Esselin
Translation Semantix
Photo Magnus Bergström
Einstein's general relativity theory
Describes what gravitation is based on three basic principles: gravitation is not a force but an effect of space-time being curved; all free objects move along the shortest possible path in this curved space; the curvature of space is an effect of mass and energy.
Complex systems
A complex system is characterized by the fact that many independent entities interact, spontaneous self-organization, and adaptive ability.
Algebraic geometry
A branch of mathematics in which one studies geometric structures (curves and surfaces) by studying the zeros of polynomial equations with one and several variables.